Some Comparing lemmas in analysis (epsilon delta language)

lemma of "less than"

Content:

\(x, y \in \Bbb{R}\), \(\forall \epsilon \gt 0\), \(x \lt y + \epsilon\), then \(x \leq y\)

Proof

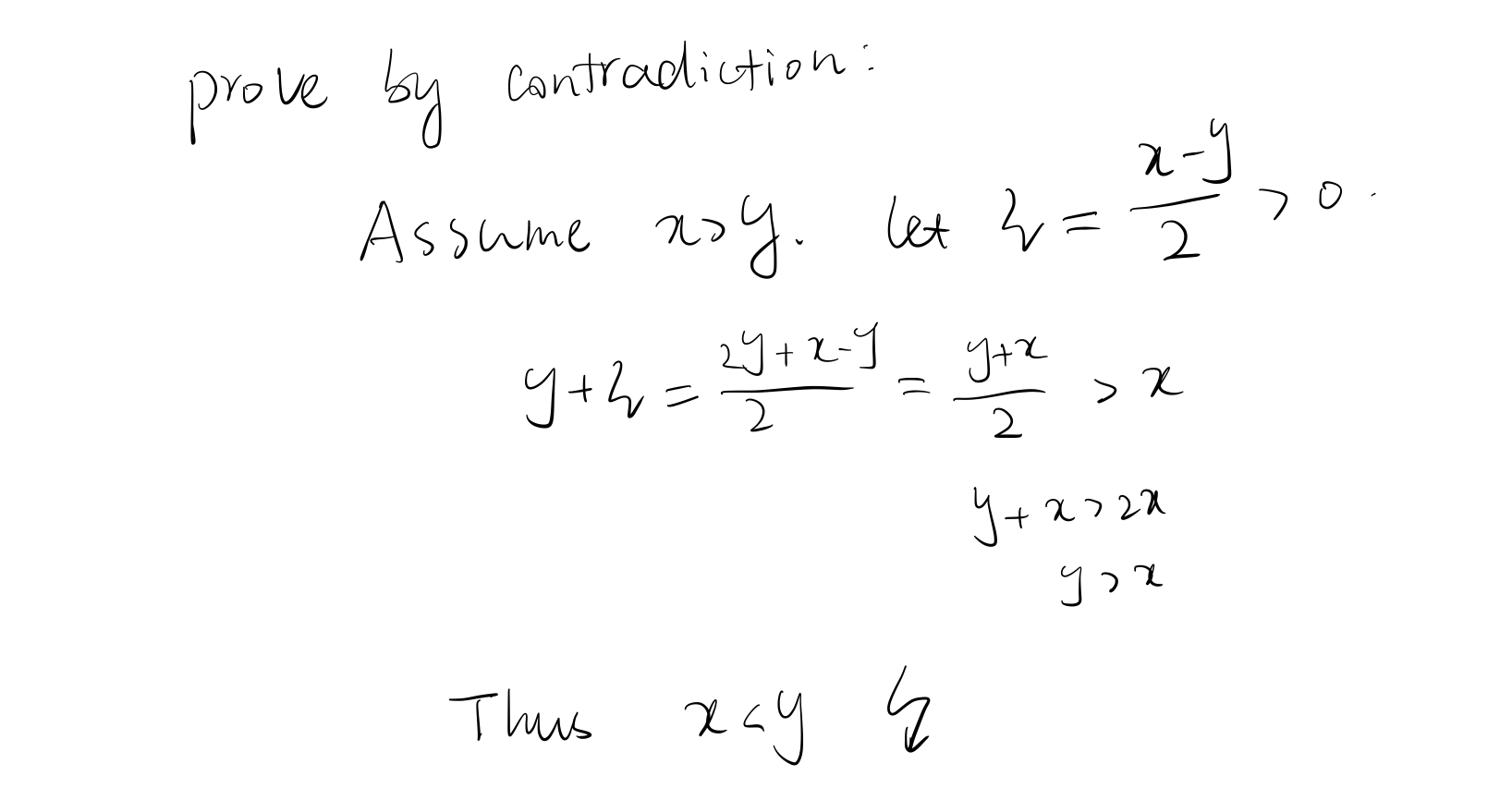
lemma of "Equal"

Content:

\(x, y \in \Bbb{R}\), \(\forall \epsilon \gt 0\), \(x = y + \epsilon\), then \(x = y\)

Proof